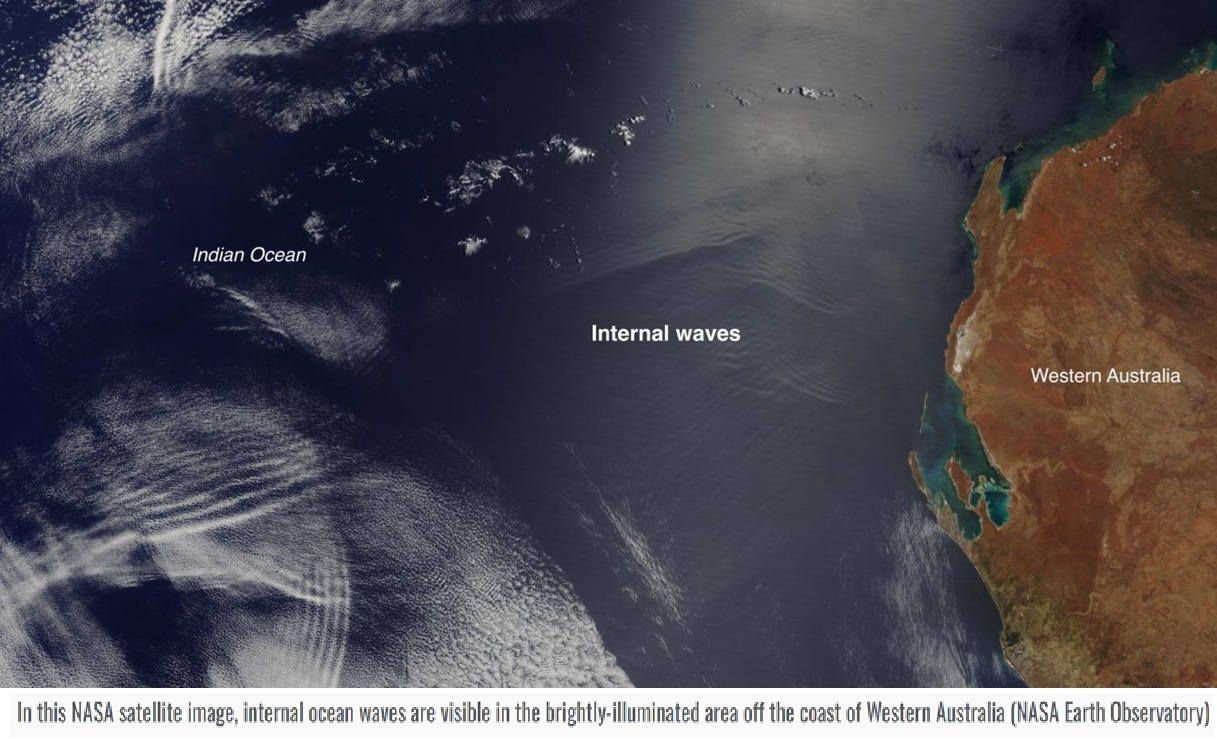
Internal waves and ocean mixing
The rate of diapycnal mixing, largely due to internal-wave breaking, is a key ingredient to understanding upwelling and horizontal circulation in the abyssal ocean. I work on developing a first-principles analysis of the downscale energy flux in the internal wave field, with the aim of providing a solid theoretical background to the finescale parameterizations that are used to include the effects of internal waves in the Ocean Global Circulation Models.